

This research project is supported by CREST - a funding program, which is run by the Japan Science and Technology Agency (JST). The aims of JST are to promote and encourage the development of breakthrough technologies that contribute to attainment of the country’s strategic objectives, and to return the results of such research to the society through innovations.
CREST supports team-oriented research with the aim of achieving the strategic goals set forth by the government. The objective is to create revolutionary technological seeds for science and technology innovation. Detailed information can be found on the JST and CREST websites。
| Research Field | Construction of new mathematical techniques in the context of modern mathematical sciences |
|---|---|
| Project Title | Mathematical Modelling for Next-Generation Cryptography |
| Principal Investigator | Tsuyoshi Takagi, Professor, Graduate School of Information Scienceand Technology, The University of Tokyo |
| Co-Investigators | Masato Wakayama, Fundamental Mathematics Research Principal,NTT Communication Science Laboratories Keisuke Tanaka, Professor, Graduate School of Information Scienceand Engineering, Tokyo Institute of Technology Noboru Kunihiro, Professor, Faculty of Engineering, Information and Systems, University of Tsukuba |
Historically, cryptography provides a good example for applications of number theory - before being applied to cryptography, this theory was believed to have no industrial applications. Currently, the newly developed technologies such as quantum computation or power analysis bring up new security risks for the modern cryptosystems. For addressing these risks, high-performance next-generation cryptosystems must be studied that will require to further develop the mathematical background of modern cryptography. More specifically, in order to avoid the security risks posed by the adversaries with advanced attacking capabilities, we must upgrade our cryptosystems, which will in turn require us to rely on a wide range of mathematical theories.
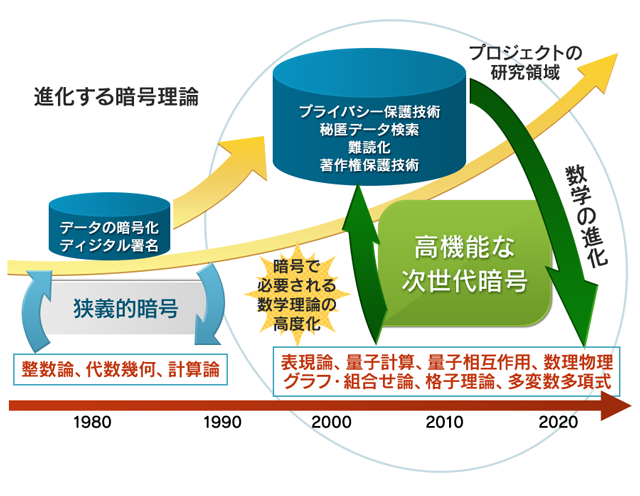
In the recent years, cryptography became concerned with questions beyond secure information transmission, such as privacy-preserving computations and search over encrypted data.
In the 1980-ies and 1990-ies, the major research topics concerned encryption and digital signatures. Then, in the 1990-ies and 2000-s, the interest has shifted towards elliptic curve cryptography, bilinear pairing based cryptography, ID-based encryption, and copyright protection technologies. Then recently, cryptographic techniques found their applications in privacy-preserving technologies, search over private data, obfuscation and others.
In order to address the challenges posed by these new applications, one must rely on a wide range of mathematical theories including representation theory, lattice theory, mathematical physics and others, both for construction of the cryptographic schemes and for their security evaluation. Therefore, one must drastically diversify the range of mathematical theories on which the modern cryptography relies.
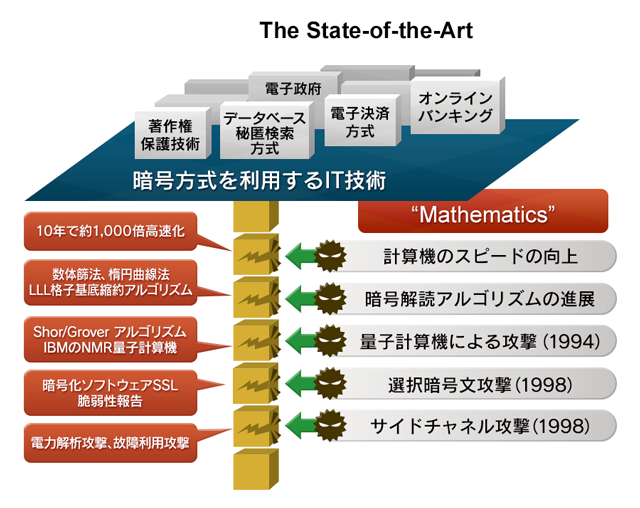
The techniques for cryptanalysis have been developing year-by-year based on the advances in mathematics, hereby permanently posing compromise risks for the employed cryptographic systems. Since 1980-ies, the algorithms such as number field sieve, elliptic curve method, Grobner bases, LLL lattice reduction algorithm and others have been developed and improved, and this process continues presently. In 1994, Shor has introduced a breakthrough algorithm which can solve the factorization and the discrete logarithm problems using the quantum computer.
This showed that in an era with large-scale quantum computers, the security of currently used cryptosystems (such as RSA, elliptic curve cryptography, pairing-based cryptography and some others) will be compromised. In order to avoid these security risks, an adequate security model must be introduced, that would encompass advances in both the cryptanalytic algorithms and the computation technologies.
This research project is focused on the study of the mathematical problems underlying a security modeling of the next-generation cryptography. We will introduce new mathematical results in order to strengthen information security, simultaneously making fresh insights and developing the respective areas of mathematics. The impact of this study will be to define new mathematical models which will helps us to ward off the recently introduced security threats. This new models will serve as guidelines for development and security assessment of the next-generation cryptographic constructions. The novel mathematical techniques and their applications, which will be brought up as a result of this study, will raise awareness of the research community about these new fields of industrial mathematics, that in turn will open up new career opportunities for mathematicians.